The results are out and this years Nobel Prize in Physics 2016 has been awarded to two different research groups; David J. Thouless with the other half going to F. Duncan M. Haldane and J. Michael Kosterlitz, ”for theoretical discoveries of topological phase transitions and topological phases of matter.”
Many expected the prize to be given to the team behind this years monumental announcement of the discovery of gravitational waves using the LIGO detector, however the Royal Swedish Academy of Sciences choose three laureates who have used advanced mathematical methods to study unusual states or phases, of matter, such as superfluids, superconductors or thin magnetic films.
Their discoveries have been stated as “bringing about breakthroughs in the theoretical understanding of matter’s mysteries and creating new perspectives on the development of innovative materials.” In the accompanying press release issued with the decision, the Academy further states, “thanks to their pioneering work, the hunt is now on for new and exotic phases of matter. Many people are hopeful of future applications in both materials science and electronics.”
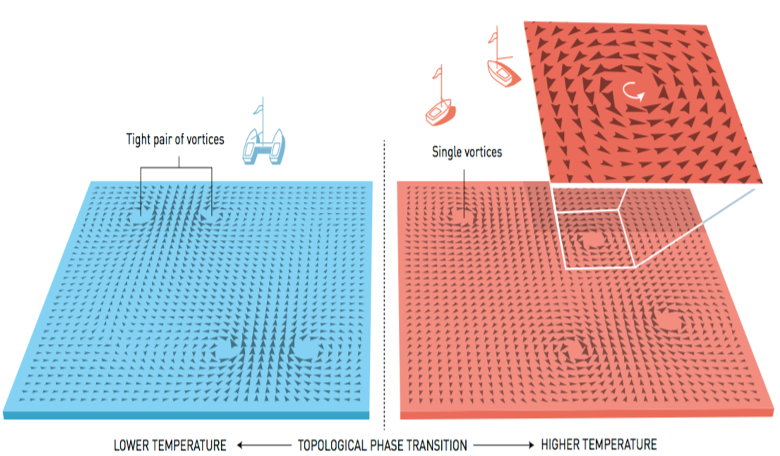
Topology, the tool used by the three laureates to astound the experts, is a branch of mathematics that describes properties that only change step-wise. In the early 70s, David J. Thouless now at the University of Washington, USA and J. Michael Kosterlitz now at Brown University, USA, pioneered work that showed that superconductivity or suprafluidity could occur in a flat world – on surfaces or inside extremely thin layers that can be considered two-dimensional – the opposite to what was currently thought at the time. They also demonstrated that superconductivity could occur at low temperatures and also explained the mechanism, phase transition, that makes superconductivity disappear at higher temperatures.
A decade later Duncan Haldane now at Princeton University,USA, discovered how topological concepts can be used to understand the properties of chains of small magnets found in some materials. Haldane has also studied matter that forms threads so thin they can be considered one-dimensional.
Over the last ten years, research into topological phases, of which many are now known, has boosted frontline research in condensed matter physics and it is hoped that topological materials can be used in new generations of electronics and superconductors, or in future quantum computers.